How to Solve 2 Step Equations with Variables
Understanding the Basics of Two-Step Equations
Mathematics can sometimes feel overwhelming, especially for those who are just beginning to explore algebra. One concept that many students—and even adults—find particularly tricky is the two-step equation. These are algebraic equations that cannot be solved in a single move; instead, they require two distinct mathematical operations to find the value of the unknown variable. Essentially, solving a two-step equation means reversing the operations that have been performed on the variable, in the correct order. Typically, this involves undoing any addition or subtraction first, followed by reversing multiplication or division. For example, in the equation 2x + 3=11, you would start by subtracting 3 from both sides to simplify the equation, and then divide by 2 to solve for x. Understanding the proper sequence and logic behind each step is key to mastering this foundational algebra skill.
What are 2-step equations?
Two-step equations are a type of algebraic equation that can be solved by performing two mathematical operations. These equations are called "two-step" because it takes exactly two steps to isolate the variable and find its value. The general form of a two-step equation looks like this:
ax + b = c
where:
a is the coefficient of the variable,(a number in front of the variable is a coefficient)
x is the variable,
b is a constant term,
c is the constant on the other side of the equation.
Here are some examples of two-step equations
2x + 3 = 11
5y - 2 = 13
4a + 7 = 19
3b - 6 = 9
What are inverse operations?
Before we delve into the specifics of how to solve 2-step equations, we need first to understand what an inverse operation means. Put simply, an inverse operation is an operation that reverses the effect of another operation. In other words, inverse operations are operations that "undo" or "reverse" each other. In mathematics, these operations are used to isolate variables and solve equations. The most common inverse operations are addition and subtraction, multiplication and division. For instance, addition and subtraction are considered inverse operations, in the same way that multiplication and division are. When you add a number and then subtract the same number, you land back to the initial value, demonstrating how these operations are "inverse". The same principle applies to multiplication and division. In summary, the inverse of addition is subtraction. The inverse of subtraction is addition. The inverse operation of multiplication is division. The inverse operation of division is multiplication. These concepts are fundamental to algebra and are often used to manipulate equations and expressions to solve problems and equations correctly.
3. Finally, undo the multiplication or division using inverse operations as described above. If the variable you are solving for is being multiplied by a number, divide both sides by this number.
This process, when carried out correctly, will give you the value of the variable. Now that we have described the steps of solving two step equations, let's consider some examples for a clearer understanding of two-step equations
How to solve 2-step equations?
Here's a step-by-step guide on how to solve a two-step equation with variable(s). The process of solving a two-step equation often involves addition/subtraction and multiplication/division. Regardless of the specific equation, the fundamental approach remains the same:
1. Start by simplifying the equation as much as possible. If there are like terms on both sides, combine them.
For example,
5x - 2x = 7
Combining like terms in this equation is subtracting 2x from 5x.
5x - 2x = 3x
3x = 7

Click here for combining like terms worksheet. Students must know how to combine like terms to solve equations.
2. Next, undo the addition or subtraction using inverse operations. Depending on the equation, this involves either adding or subtracting on both sides of the equal sign.
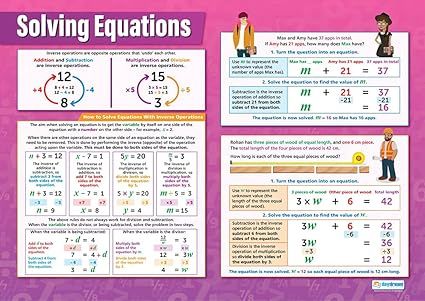
combining like terms worksheet pdf click here
inverse operations poster click on pic to buy
Examples of Two-Step Equation Problems and Solutions
Example 1: Solve for x in the equation 2x + 3 = 9.
Step 1: Subtract 3 from both sides to undo the addition. Subtraction is the inverse operation of addition.
.png/:/cr=t:0%25,l:0%25,w:100%25,h:100%25/rs=w:1280)
Step 2: Divide by 2 on both sides to undo the multiplication
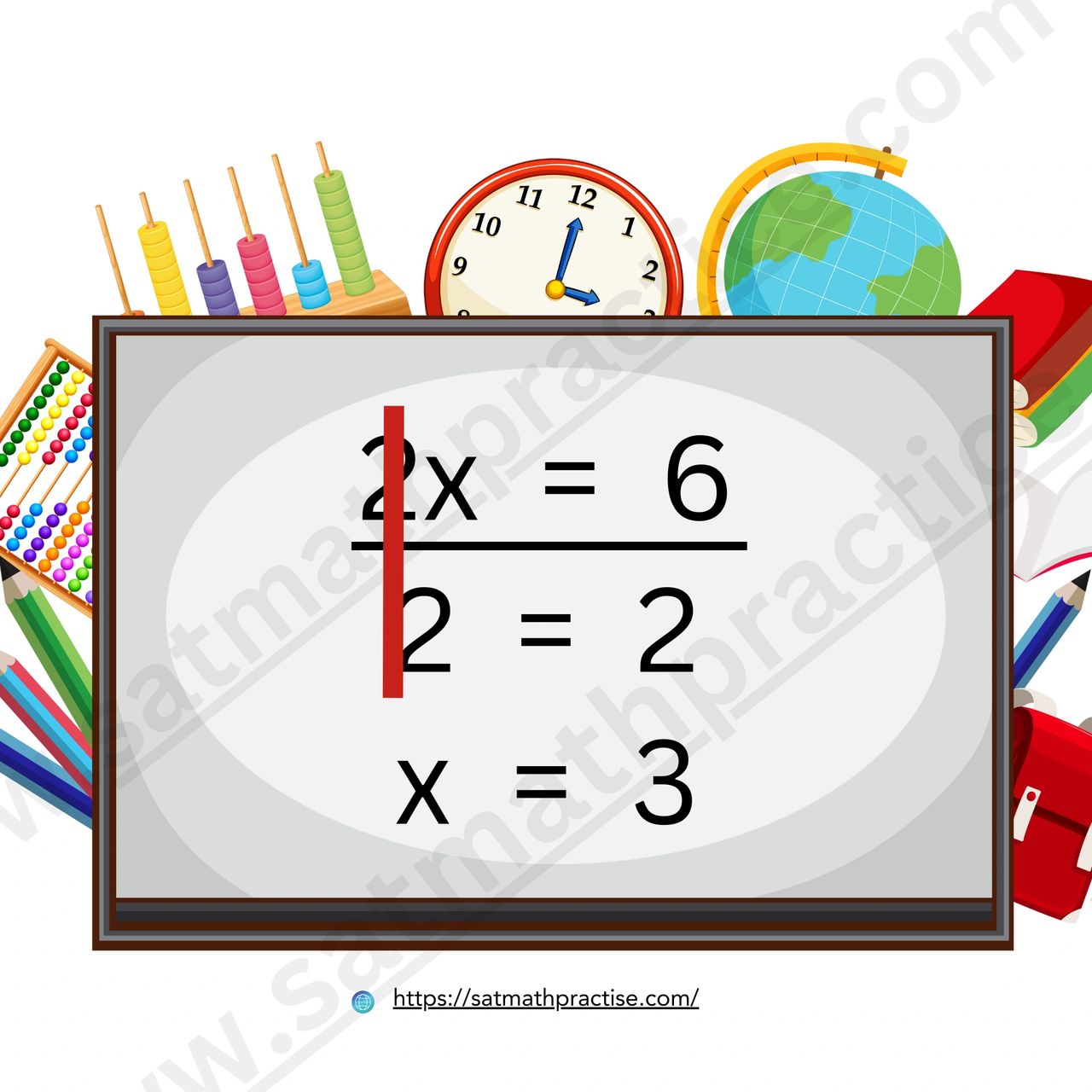
Example 2: Solve for y in the equation 3y - 7 = 2.
Step 1: Add 7 to both sides to undo the subtraction
3y - 7 + 7 = 2 + 7 =>
3y = 9
Step 2: Divide by 3 on both sides to undo the multiplication
3y / 3 = 9 / 3 =>
y = 3
Common Mistakes to Avoid When Solving Two-Step Equations
When solving two-step equations, it's common for learners to make a few mistakes that can lead to incorrect answers. Understanding these errors can help you avoid them and improve your problem-solving skills. Here are some of the most frequent mistakes:
Misapplying the order of operations:
One of the most common mistakes is confusing the correct order in which to undo operations. In two-step equations, you are not following the standard order of operations (PEMDAS) used to evaluate expressions. Instead, you must reverse those operations to isolate the variable. That means you should undo addition or subtraction first, and then multiplication or division. For example, in the equation 4x + 7 = 19, the correct approach is to subtract 7 before dividing by 4. Doing the steps out of order leads to incorrect solutions.Not applying the same operation to both sides of the equation:
Equations are like balanced scales—whatever you do to one side must also be done to the other to keep it balanced. Some learners forget this and only change one side of the equation, breaking the equality. For example, if you subtract 7 from one side but not the other, the equation becomes unbalanced and the solution will be incorrect. Always apply operations to both sides of the equation.Skipping simplification before solving:
Before starting the two-step process, it’s important to simplify the equation as much as possible. This includes combining like terms and eliminating unnecessary parentheses when possible. If you try to solve without simplifying first, you may end up working with a more complicated version of the equation, increasing the chance of errors. For example, in 2x + x + 3 = 12, you should first combine 2x+x to get 3x + 3 = 12, and then proceed with solving.
By recognizing and avoiding these common mistakes, you'll become more accurate and confident when solving two-step equations.
2 step equations practice
Now that you have a good understanding of two-step equations, it's time to apply this knowledge and challenge yourself with two-step equations:
1. Solve x + 5 = 2x - 7.
2. Solve for y in the equation 3y - 11 = 5.
3. Solve for z in the equation 4z + 6 = 18.
Remember to practice and reinforce what you've learned. The more two-step equation problems you solve, the more adept you will become at handling them. Happy solving!
Challenge answers: 1) x = 12 2) y = 5.33 or 5 1/3 3) z = 4
Now that you have practiced and have your answers to the challenge questions, how can you check your answer to your 2-step equations? To verify your answers in 2-step equations, simply plug your solution back into the initial equation to determine if both sides are equal. Acquiring the ability to self-check your answers in algebra will help you become more successful in all of your math classes.
solving two-step equations worksheet doc

2 step equations and multi-step equations worksheet
FTC Disclaimer: This post may contain affiliate links, We may earn a small commission from qualifying purchases when you click a link at no extra cost to you. As an Amazon Associate, I earn from qualifying purchases at no cost to you.
